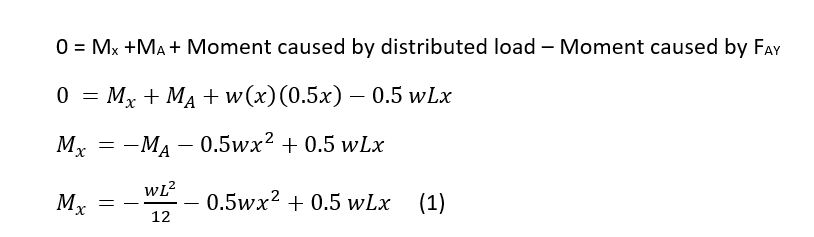
As described in previous blog posts about Near Infrared Spectroscopy (NIRS), NIRS is a valuable tool to measure oxygen concentration in the body. It can be used on various parts of the body including the muscles and brain. These applications of NIRS to measure oxygen concentration is useful in metabolic kinetics research, diagnoses of disease conditions [1], and as an athletic performance measure. Recent advancements in NIRS technology have allowed for the development of portable NIRS devices that can be worn while exercising. In this post, I will be focusing on the use of NIRS technology for the measurement of muscle oxygenation.
The main benefit of NIRS is that it can measure oxygen concentration, as discussed above. In order to do this the use of spectroscopy methodology is used. Spectroscopy is when light of a specific wavelength is transmitted through a substance and the amount of light the substance absorbs is measured and called the absorbance. From the absorbance, the concentration of a solute can be determined using Beer-Lambert’s Law. The Beer-lambert’s Law relates the light intensity to the product of the molar absorptivity (ε, L/mol*m), the substance concentration (c, mol/L), and the path length (L, m). Due to the path length not being perfectly defined, a modified version of the Beer-Lambert’s Law is used (Equation 1) that accounts for any scattering (g) of the light beam as it travels through the tissue. The initial intensity of the light (I) then is the sum of the light that is reflected, the light that is transmitted to the detector and the light that is scattered throughout the tissue.
log10(I0/I) = εcL+g (1)
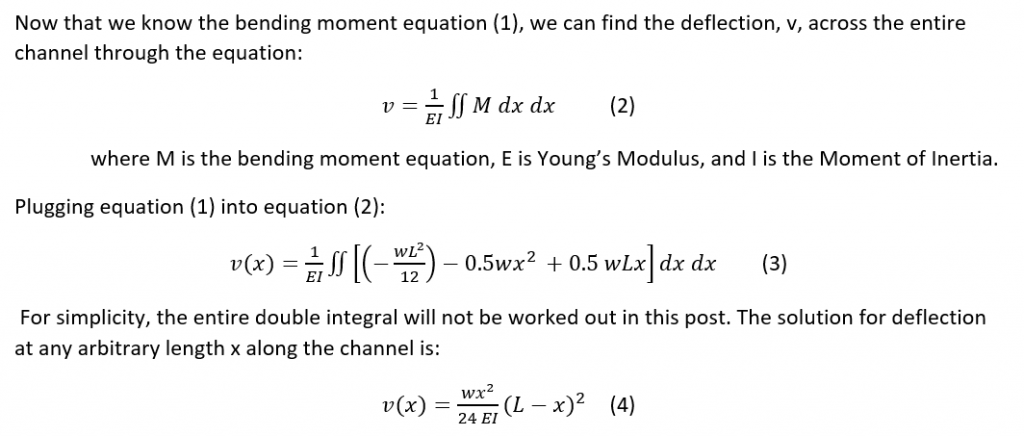
As I have outlined, the main function of NIRS for measuring muscle oxygenation is to be able to measure the absorbance of the hemoglobin in the blood. To do this the right wavelengths must be able to penetrate the body and get picked up by the detector where the absorbance can be measured. By solving the problem of what wavelength of light to use, the device can effectively function for its purpose and be reliable. The wavelength of light appropriate depends on what is being looked at. In the case of NIRS, the absorbance of hemoglobin in the blood is the target substance. When oxygen is bound to hemoglobin (oxyhemoglobin), the hemoglobin has a different absorbance value (Figure 1) than if there is no oxygen bound to it (deoxyhemoglobin). The question is: what is the appropriate wavelength to use?
Figure 1. Absorbance curves for oxyhemoglobin (red) and deoxyhemoglobin (blue).
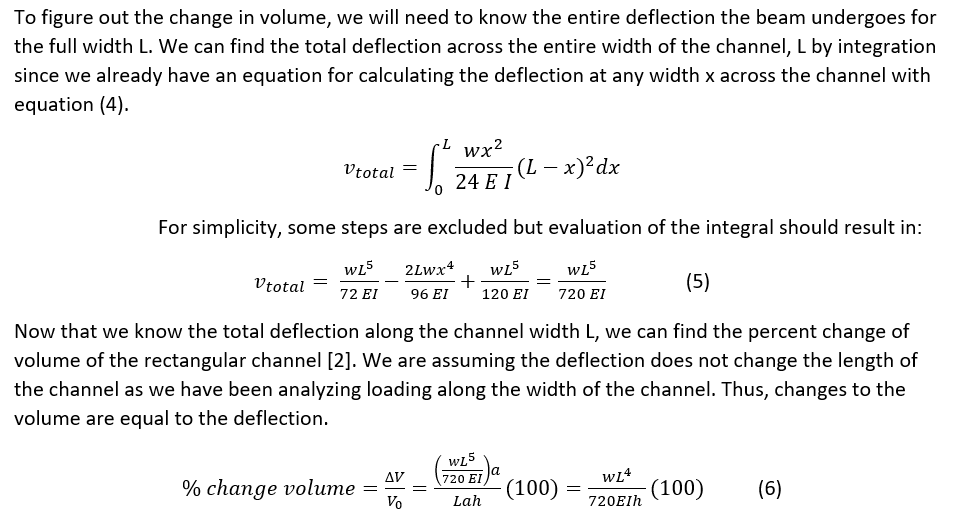
In order to work through and solve the problem of what wavelength to use, we must first consider what is known and what is unknown. Referring to Equation 1, the modified Beer-Lambert Law, the absorptivity is a known variable dependent on the solute looking at. Another known variable is the path length that can be determined by measuring the length between the light source or probe and the detector surface. That leaves the solute concentration and the scattering term as the unknowns. In order to solve the equation for concentration and determine the optimal wavelengths for NIRS device functionality, the scattering term needs to be eliminated from the equation. In order to do this, we need to realize that the scattering term is very variable; it depends on the subject, the location, and muscle the NIRS device is being used on. For the device to be used by a larger population the scattering term must be normalized and this is accomplished by finding the change in concentration. This is why concentrations are reported not in absolute concentrations but in relative measures, percentages. By subtracting two absorbances (change in absorbance), the scattering terms will cancel out assuming the scattering term is the same over one location. Now the equation for change in concentration is:
log10(I0/I) = εΔcL (2)
Now when we account for the fact that NIRS measures not only oxyhemoglobin concentrations but also deoxyhemoglobin concentrations we need to expand our equation to account for both. That means that the change in concentration is the change in concentration of oxyhemoglobin and the change of concentration in deoxyhemoglobin.
log10(I0/I) = (εO2ΔcO2 + εHbΔcHb)L (3)
When we add another unknown thought we need to generate another equation to be able to solve for the two unknown concentrations leaving us with the following equations, Equation 4.

https://cdn.iopscience.com/images/0031-9155/51/5/N02/Full/pmb211409eqn04.gif
Now that we have equations where the subscripts λ1 and λ2 are the two wavelengths [2] and the subscripts O2 is for oxyhemoglobin and Hb is for deoxyhemoglobin. There is one unknown in each so we can now determine a wavelength that will be optimal for both oxyhemoglobin and deoxyhemoglobin so the NIRS device will be able to measure oxygen concentration in the muscles. Solving the equations so that one is sensitive to oxyhemoglobin, and one is more sensitive to deoxyhemoglobin, we come up with the optimal range of 0.7 um to 2.5 um. The near infrared region of light. This is reasonable and coincides with what research says that near infrared light is the optimal waveforms to have light waves penetrate the muscles and measure oxygen concentrations [3].
In this solution, we made the assumption that the scattering term was the same in the same location but that is not necessarily the truth. If the light was angled differently when it was absorbed, there could be different amounts of scatter even in the same location. Without this simplification, the equation would be much more complex because it would have to take into consideration all factors that affect the scattering of light waves in the tissue which would require frequent calibrations and mathematical adjustments. Now that we have answered the question of what wavelengths are optimal for the NIRS to measure oxyhemoglobin and deoxyhemoglobin concentrations we can start measuring concentration!
References:
[1] Adami A, Rossiter HB. Principles, insights, and potential pitfalls of the noninvasive determination of muscle oxidative capacity by near-infrared spectroscopy. J Appl Physiol (1985) 124: 245–248, 2018. doi:10.1152/japplphysiol.00445.2017. https://journals.physiology.org/doi/full/10.1152/japplphysiol.00445.2017
[2] “About NIRS (Principle of Operation and How It Works),” About NIRS (Principle of Operation and How It Works) | SHIMADZU EUROPA. [Online]. Available: https://www.shimadzu.eu.com/about-nirs-principle-operation-and-how-it-works. [Accessed: 13-May-2020].
[3] L. Kocsis, P. Herman, and A. Eke, “The modified Beer–Lambert law revisited,” Physics in Medicine and Biology, vol. 51, no. 5, 2006.




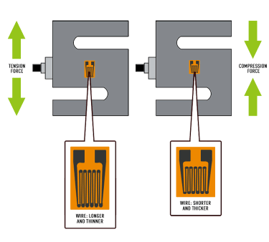



 With resistivity, original length of wire be constants and cross-sectional area changed being assumed miniscule, so also constant for simplicity
With resistivity, original length of wire be constants and cross-sectional area changed being assumed miniscule, so also constant for simplicity